power series
mathematics
in mathematics, an infinite series that can be thought of as a polynomial with an infinite number of terms, such as 1+x+x2+x3+⋯. Usually, a given power series will converge (convergence) (that is, approach a finite sum) for all values of x within a certain interval around zero—in particular, whenever the absolute value of x is less than some positive number r, known as the radius of convergence. Outside of this interval the series diverges (is infinite), while the series may converge or diverge when x=±r. The radius of convergence can often be determined by a version of the ratio test for power series: given a general power series
a0+a1x+a2x2+⋯,
in which the coefficients are known, the radius of convergence is equal to the limit of the ratio of successive coefficients. Symbolically, the series will converge for all values of x such that
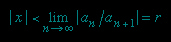
For instance, the infinite series 1+x+x2+x3+⋯ has a radius of convergence of 1 (all the coefficients are 1)—that is, it converges for all −1\\<x\\<1—and within that interval the infinite series is equal to 1/(1−x). Applying the ratio test to the series
1+x/1!+x2/2!+x3/3!+⋯
(in which the factorial notation n! means the product of the counting numbers from 1 to n) gives a radius of convergence of
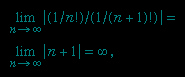
so that the series converges for any value of x.
 Most functions can be represented by a power series in some interval (see table-->
Most functions can be represented by a power series in some interval (see table--> ). Although a series may converge for all values of x, the convergence may be so slow for some values that using it to approximate a function will require calculating too many terms to make it useful. Instead of powers of x, sometimes a much faster convergence occurs for powers of (x−c), where c is some value near the desired value of x. Power series have also been used for calculating constants such as π and the natural logarithm base e and for solving differential equations (differential equation).
). Although a series may converge for all values of x, the convergence may be so slow for some values that using it to approximate a function will require calculating too many terms to make it useful. Instead of powers of x, sometimes a much faster convergence occurs for powers of (x−c), where c is some value near the desired value of x. Power series have also been used for calculating constants such as π and the natural logarithm base e and for solving differential equations (differential equation).- Hassel, Odd
- Hasselt
- Hasselt, André van
- Hassenpflug, Hans Daniel
- Hassi Messaoud
- Hassi RʾMel
- hassium
- Hassler, Hans Leo
- Hasso, Freiherr von Manteuffel
- Hasso Manteuffel, Freiherr von
- Hass, Robert
- Hassuna
- Hastings
- Hastings, Battle of
- Hastings, Francis Rawdon-Hastings, 1st marquess of
- Hastings, Frank Abney
- Hastings Kamuzu Banda
- Hastings Lionel Ismay, Baron Ismay
- Hastings Lionel Ismay Ismay, Baron
- Hastings, Warren
- Hastings, William Hastings, Baron
- hat
- Hat Act
- Hatano Seiichi
- Hata Tsutomu