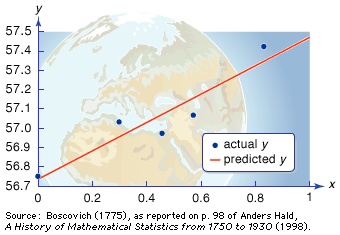To settle the dispute, in 1736 the French Academy of Sciences (Sciences, Academy of) sent surveying expeditions to Ecuador and Lapland. However, distances cannot be measured perfectly, and the measurement errors at the time were large enough to create substantial uncertainty. Several methods were proposed for fitting a line through this data—that is, to obtain the function (line) that best fit the data relating the measured arc length to the latitude. It was generally agreed that the method ought to minimize deviations in the y-direction (the arc length), but many options were available, including minimizing the largest such deviation and minimizing the sum of their absolute sizes (as depicted in the figure-->). The measurements seemed to support Newton's theory, but the relatively large error estimates for the measurements left too much uncertainty for a definitive conclusion—although this was not immediately recognized. In fact, while Newton was essentially right, later observations showed that his prediction for excess equatorial diameter was about 30 percent too large.