orthogonal trajectory
mathematics
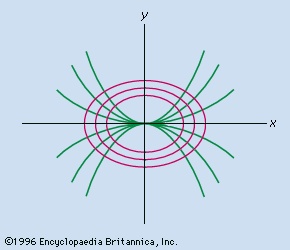 family of curves that intersect another family of curves at right angles (orthogonal; see figure-->
family of curves that intersect another family of curves at right angles (orthogonal; see figure--> ). Such families of mutually orthogonal curves occur in such branches of physics as electrostatics, in which the lines of force and the lines of constant potential are orthogonal; and in hydrodynamics, in which the streamlines and the lines of constant velocity are orthogonal.
). Such families of mutually orthogonal curves occur in such branches of physics as electrostatics, in which the lines of force and the lines of constant potential are orthogonal; and in hydrodynamics, in which the streamlines and the lines of constant velocity are orthogonal.In two dimensions, a family of curves is given by the function y=f(x,k), in which the value of k, called the parameter, determines the particular member of the family. Two lines are orthogonal, or perpendicular, if their slopes are negative reciprocals of each other. Curves are said to be perpendicular if their slopes at the point of intersection are perpendicular. Depending on context, the slope may also be called the tangent or the derivative, and it can be found using differential calculus (analysis). This derivative, written as y′, will also be a function of x and k. Solving the original equation for k in terms of x and y and substituting this expression into the equation for y′ will give y′ in terms of x and y, as some function y′=g(x,y).
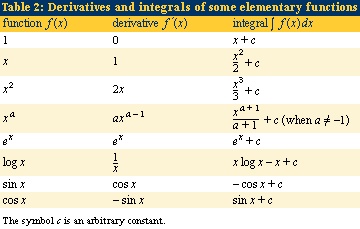 As noted above, a member of the family of orthogonal trajectories, y1, must have a slope satisfying y′1=−1/y′=−1/g(x, y), resulting in a differential equation that will have the orthogonal trajectory as its solution. To illustrate, if y=kx2 represents a family of parabolas (parabola), then y′=2kx (see the table-->
As noted above, a member of the family of orthogonal trajectories, y1, must have a slope satisfying y′1=−1/y′=−1/g(x, y), resulting in a differential equation that will have the orthogonal trajectory as its solution. To illustrate, if y=kx2 represents a family of parabolas (parabola), then y′=2kx (see the table--> of common derivative rules from analysis), and, because k=y/x2, a substitution of the latter in the former yields y′=2y/x. Solving this for the orthogonal curve gives the solution
of common derivative rules from analysis), and, because k=y/x2, a substitution of the latter in the former yields y′=2y/x. Solving this for the orthogonal curve gives the solution y2+(x2/2)=k,
which represents a family of ellipses (ellipse) orthogonal to the family of parabolas.
- Lost Generation
- lost-wax process
- Lostwithiel
- Lota
- Lot, Ferdinand
- Lothagam
- Lothar
- Lothar Bucher
- Lothar I
- Lothar (II)
- Lothar II (or III)
- Lothar Matthäus
- Lothar Meyer
- Lothar von Faber
- Lothian
- Lotichius Secundus, Petrus
- Loti, Pierre
- Lot River
- Lotta Crabtree
- Lotte Jacobi
- Lotte Lehmann
- Lotte Lenya
- lottery
- Lotto carpet
- Lotto, Lorenzo