expression of a number as the sum of an integer and a quotient, the denominator of which is the sum of an integer and a quotient, and so on. In general,

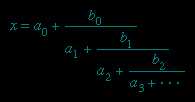
where a₀, a₁, a₂, … and b₀, b₁, b₂, … are all integers.

In a simple continued fraction (SCF), all the b_i are equal to 1 and all the a_i are positive integers. An SCF is written, in the compact form, 【a₀; a₁, a₂, a₃, …】. If the number of terms a_i is finite, the SCF is said to terminate, and it represents a rational number; for example, ⁸⁰²/₂₅₁=【3; 5, 8, 6】. If the number of these terms is infinite, the SCF does not terminate, and it represents an irrational number; for example, √23=【4; 1, 3, 1, 8】, in which the bar spans a sequence of terms that repeats indefinitely. A nonterminating SCF in which a sequence of terms recurs represents an irrational number that is a root of a quadratic equation with rational coefficients. Nonterminating SCFs that represent numbers such as π or e can be evaluated after any given number of terms to obtain a rational approximation to the irrational quantity.